⿻ Voting

⿻ Voting

In the best-selling strategy game of all time, Civilization VI, players manage a civilization from the birth of the first settlements to the near future, competing and sometimes cooperating with other civilizations in a race to victory through culture, military conquest, diplomatic support, scientific achievement and/or religious influence. In the game's widely adopted and climate change-themed expansion pack "Gathering Storm", diplomatic decisions affecting the whole world are decided in a "World Congress". Civilizations accumulate "diplomatic favor" from alliances, infrastructure, and so forth. They can then spend these to influence global policies, such as regulation of fossil fuels, controls on nuclear weapons or immigration rules.

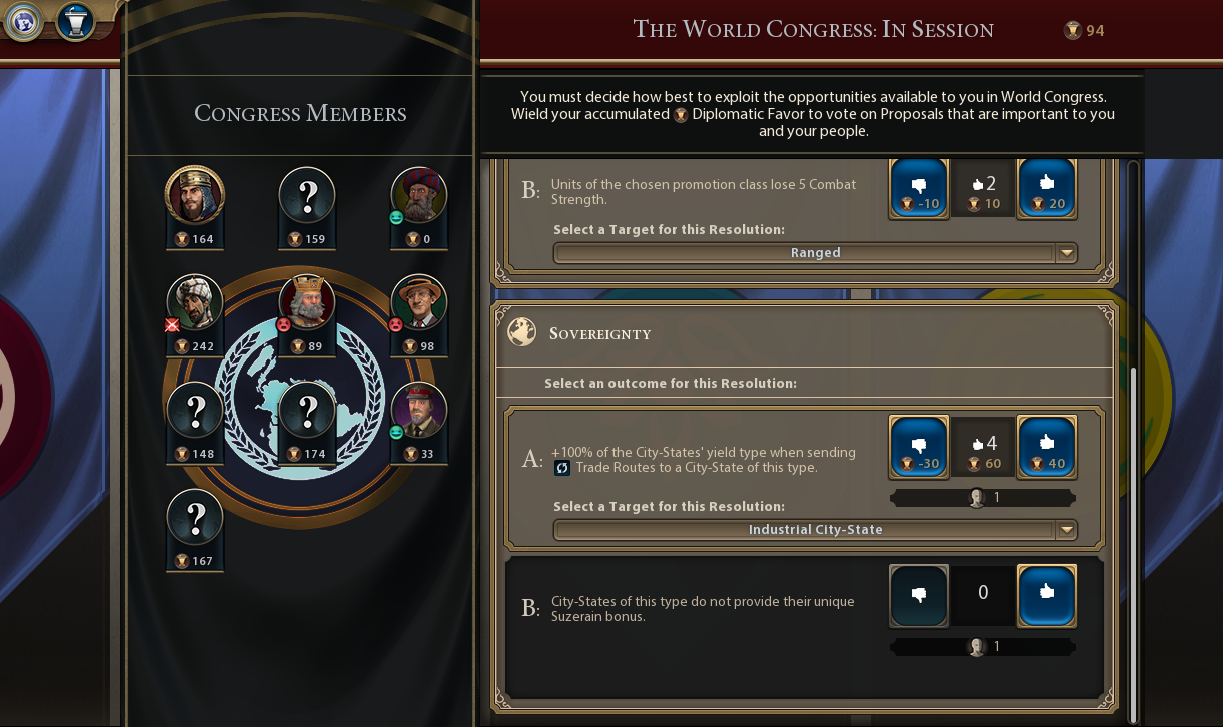
When voting, countries can choose from a range of options, such as which civilization will be targeted for closer scrutiny of its actions by the world. Every civilization gets a single vote for free, but additional votes cost increased diplomatic favor, at an increasing rate. The first additional vote costs 10 diplomatic favor, the second 20, and so on, as illustrated in Figure A. There are typically several votes on different issues in a single Congress and diplomatic favor can be saved across Congresses as well as used for other purposes such as nominating special issues for consideration. Each civilization must thus gauge how important each issue is to it. Then "buy" votes using diplomatic favor just up to the point where the amount they care matches the increasing cost of having more influence on that issue compared to the value of saving their favor.

This game mechanic is a variant of the "quadratic voting" procedure one of us invented, which is now widely used outside of games as well, as we will explore below.^[1] Because of the logic above, it aggregates not just the direction of individual preferences but also their strength. Thus, when individual action is independent, it can lead to decisions based not just on "the greatest numbers" but "the greatest good for the greatest number".

A main theme of this part of the book has been how much broader collaborative technologies and democracy are than the institutions we might usually associate with them. Yet, the formal institutions that most come to mind when we think about "democracy" are systems for holding votes and elections. Voting is used throughout not just democratic systems, but governance regimes more broadly: corporate governance, management of cooperative housing, book clubs, games etc. It provides a way for a large and diverse group to, relatively quickly and at relatively low cost, make a definite decision on a point of disagreement. While the communication it allows is far thinner than the technologies we have thus far described, it can often be a much more broadly inclusive process that leads to verdicts of the "common will" that are typically thought of as more legitimate (at least among the usually limited set of those enfranchised) than the outcomes of markets. In this chapter, we will explore the ways voting works and fails to work in the settings it is most often applied today, innovations like quadratic voting (QV) that are creating higher-fidelity signals of the "public will", and peer into the horizon of ways researchers are re-imagining how large groups of people can choose their future together.

Voting today

In the most common form of voting, every member of some community selects one of several mutually exclusive options and the option with the most votes is selected. Some trace this practice to the ability of a group with greater numbers to triumph in certain kinds of violent conflicts (such as phalanx engagements in Ancient Greece), which could be avoided by tallying the strength of positions. Despite its simplicity, this "plurality rule" is not a particularly compelling representation of ⿻ in the way we use it, for several reasons including:

1. It tends to create a "lesser of two evils" dynamic (known as "Duverger's Law" to political scientists) where people are forced to vote for one of the two leading alternatives even if they dislike both and some trailing alternative might win broader support.^[2]
2. In many contexts, the simple equality assumed in such a tally is not widely legitimate. Different participants in a vote may have differing degrees of legitimate interest in an issue (e.g. representing different populations, having spent longer time in a community, etc.).
3. Even at its best, it represents the direction in which a majority chooses, rather than an overall sense of the "will of the group", which should include how important different issues are to people and how much they know about them. This is often called the "tyranny of the majority".

A range of widely used voting procedures aim to address these challenges in limited ways such as:

• Ranked choice and approval voting: These two recently popular systems partially address issue 1. In ranked choice systems, participants rank a number of alternatives, and the decision depends on this full list in some way. The simplest examples are "run-off" type systems, where the set of candidates is gradually narrowed and, as this happens, the top choice of each person for the remaining candidates becomes their new vote. In approval voting, voters may choose as many options as they wish to "approve" and the most approved option is selected. Both methods clearly have a ⿻ character both literally in allowing multiple votes and spiritually in allowing both greater consensus and greater diversity of parties by avoiding the Duverger "spoiler effect". However, economics Nobel Laureate Kenneth Arrow famously proved in his "Impossibility Theorem" that no system with such simple inputs can generally achieve a "reasonable" representation of the common will.^[3]
• Weighted voting: In contexts where equality of voters is obviously inappropriate, weighted voting schemes are used. Common examples are "one-share-one-vote" in corporate governance, voting based on population size in federal and confederal bodies (e.g. the European Union or United Nations) and voting based on measures of power (e.g. GDP) in contexts where it is thought important to respect power differences. These weights are, however, often the subject of significant dispute and lead to paradoxes of their own, such as the "51% attack" (also known as "tunneling") where someone can buy 51% of a corporation and loot its assets, expropriating the remaining 49%.^[4]
• Federal, proportional and consociational representation: While voting systems are, as we have discussed above, usually formally "monistic", there are important examples of trying to address the tyranny of the majority this can create. In federal, consociational and functional systems, sub-units, such as geographies, religions, ethnic or professional groups, have a status beyond simply their population and usually receive some kind of special or population-disproportionate weight intended to avoid oppression by larger groups. While these systems thus in various ways incorporate ⿻ elements, their design is typically haphazard and rigid, based on historical lines of potential oppression that may no longer track the relevant social issues or can entrench existing divides by formally recognizing them; they thus have become increasingly unpopular.^[5] More flexible are systems of "proportional representation", where representatives in some body are chosen in proportion to the votes they receive, helping achieve greater balance, though often at least partly "kicking the can" of majoritarian tensions down the road to the decisions of the representative body's coalition formation.

Thus, while voting is a canonical democratic technology, it is also one riddled with paradoxes, rigidity and widely recognized unsolved problems. A new generation of approaches has recently tried to improve more dramatically on what is possible.

⿻ Voting tomorrow

While the above problems seem diverse, they boil down to two questions: how to appropriately represent degrees and weights of interests and how to make representation flexible and adaptive. As Nobel Laureate Amartya Sen famously observed, problems with Arrow's Theorem vanish once strength and weight of preference is accounted for, and evidently weighted voting is all about such issues.^[6] Representation of subgroups is challenging as there are strong ⿻ reasons for doing it, yet many ways of achieving it seem insufficient or overly rigid and prescriptive. These strike at the core of the problem with the extreme simplicity of votes: they carry very limited information about voters' thoughts and preferences.

Two recent developments have offered exciting, though incomplete, approaches to addressing these problems. We highlighted the first at the start of the chapter: quadratic voting and other related approaches to incorporating voting weights. Quadratic voting originates with statistician (and, unfortunately, eugenicist) Lionel Penrose, father of the prominent contemporary astrophysicist Roger Penrose. He noted that, when weighing votes, it is natural, but misleading, to give a party with twice the legitimate stake in a decision twice the votes. The reason is that this will typically give them more than twice as much power. Uncoordinated voters on average cancel one another out and thus the total influence of 10,000 completely independent voters is much smaller than the influence of one person with 10,000 votes.^[7]

A physical analogy, prominently studied simultaneously with Penrose by J.C.R. Licklider (our hero in The Lost Dao above), may be useful to see why.^[8] Consider a noisy room where one is trying to have a conversation. It is often the case that the overall decibels of the noise are far greater than the strength of the voice of a conversation partner. Yet it is often still possible to hear what they are saying. Part of this is driven by the human capacity for focus, but another factor is that precisely what makes the background "noise" is that each contributor is far weaker than the (closer) voice one is attending to. Given that the sounds of all this noise are largely unrelated, they tend to cancel out on average and allow the one voice that is just a bit stronger to shine far stronger. Visual signal processing can be similar, where a range of scribbles fade into a gray or brown background, allowing a clear message that is only slightly stronger to stand out against it.

When background signals are completely uncorrelated and there are many of them, there is a simple way to mathematically account for this: a series of uncorrelated signals grows as the square root of their number, while a correlated signal grows in linear proportion to its strength. Thus 10,000 uncorrelated votes will weigh as heavily as only 100 correlated ones. This implies that, to award the holder of stake only proportionately greater power, its voting weight should grow as the square root of its stake, a principle often called "degressive proportionality". This in turn suggests a direction for addressing several challenges above by making a geometric (multiplicative) compromise between the intuitions of weighted and simple voting and by allowing expression of preference strength across issues and votes but taking the square root of the "weight" a voter puts on any issues. The former idea is Penrose's "square-root voting" rule, approximately used in several elements of governance in the European Union across member nations. The later is the QV rule we discussed above and used, for another example, frequently in the Colorado State Legislature to prioritize spending.

It is important to note, however, that these clean rules are only optimal when voters are perfectly internally unified and perfectly externally uncorrelated/uncoordinated. ⿻ thinking cautions us against such simplistic models, encouraging us to perceive the social connections across individuals and organizations, though of course accounting for these within a voting system requires identity systems that can record and account for these.

Another compatible approach that has gained ground in recent years is "liquid democracy"(LD). This idea, which traces back to the path-breaking work of Charles Dodgson (a.k.a. Lewis Carroll, author of the children's classic Alice in Wonderland), who also first posed the question of weighting of votes for people holding multiple votes that helped inspire QV.^[9] LD extends the idea of proportional representation, allowing any voter to delegate their vote(s) to others, who may then re-delegate them, allowing bottom-up, emergent patterns of representation.^[10] Such systems are increasingly common, especially in corporate and other for-profit (e.g. DAO) governance, as well as in a limited set of political contexts such as Iceland. However, these systems have an unfortunate tendency to concentrate power often excessively, given that delegation often flows to a small number of hands. This tendency has somewhat soured initial enthusiasm.

Frontiers of voting

The radical and transformative potential of QV and LD suggest ways that voting systems in the future may be vastly richer than those we are accustomed to. The range of possibilities are nearly endless, but a few promising ones are useful to illustrate this breadth:

• Correlation discounting and eigenvoting: QV and the Penrose rule apply degressive proportionality (using the the square-root rule) to the voting weights of respectively individuals and/or social groups (like nations). A natural extension would be to allow for a wider diversity of sources of correlation/coordination within and across individuals, as would be true in a general statistical model. In this case, an optimal rule would likely involve partial "correlation discounting" based on the degree of social connection and, perhaps, the identification of underlying "principal" social factors that drive coordination and correlation, as is common in statistical modeling.^[11] These underlying independent factors, called "eigenvalues", could then be viewed as the "real" independent voters, to whom degressive proportionality could be applied, a process not dissimilar to how PageRank works. This could create a dynamic, adaptive, optimized version of consociationalism that avoids its rigidity and entrenchment of existing divides.
• Adaptive representation: Another approach to similarly adaptive representation would be a single-member district or federal system, but with boundaries not based (exclusively) on geography but instead current social divides, such as geographic type (urban v. rural), race, or education. Clearly both this and the previous idea rely heavily on a ⿻ identity system to allow these features to be inputs into the voting process.
• Predictive voting: Robin Hanson has long advocated combining prediction markets (where people bet on future outcomes) with voting. While the "Futarchy" proposal he has advanced focuses on a cleaner separation between these two elements, in the governance of this book described above we use such a mixture, with participants being able to simultaneously vote and predict the outcome of a decision, being rewarded for a correct decision.^[12] Such systems may be particularly useful when there is a large range of proposals or options: predictions can help bring attention to proposals deserving attention that voting can then decide on.
• Quadratic liquid democracy: As noted above, a natural way to avoid the power concentrations that liquid democracy can give rise to is the use of degressive proportionality. RadicalxChange, a non-profit advancing ⿻, has implemented a related system for its internal decision-making.
• Assisted real-time voting: Another commonly discussed idea is that voting could be made far more frequent and granular if digital assistants could learn to model voters' perspectives and preferences and vote on their behalf and subject to their review/auditing.^[13]

Perhaps the most exciting possibilities are now these could combine infinite diversity, infinitely combining to support the infinite combinations that they help infinite diversity form.

Limits of ⿻ voting

One natural concern, however, about even these highly flexible and adaptive approaches to reach a sense of compromise is that the compromise itself throws the baby of diversity out with the bathwater of conflict. Yet one of the most interesting properties of systems like eigenvoting or sophisticated forms of liquid democracy is the new kinds of coalitions and representations they might help form. If one-person-one-vote rules originated from the attempt to avoid conflict by giving the side with greater support a non-violent way to take power, these systems help diffuse conflict based on a more sophisticated theory: that it arises from the consistent reinforcement of existing social divisions by allowing the same groups to consistently form majorities and minorities. By discounting support from previously affiliated groups, they avoid reinforcing existing conflicts, while creating new ones that cut across these lines, hopefully thereby generating nearly as much diversity as they compromise over, but in directions that avoid entrenching persistent divides.

Yet despite these strengths, even in its richest form, voting expresses and determines preferences about decisions already posed by other social processes. Some combination of the methods above can completely transform how we understand voting, leaving today's approaches as far behind as the computer left the abacus. Yet it would fundamentally undermine the richness of our humanity to allow this potential to fool us into believing they can substitute for the need for the richer communication and codesign we have described in previous chapters. Only in the context of the creative collaborations, deliberations, imaginations, and administrative systems we have sketched can collective decisions be meaningful.

Nor is it likely that, anytime in the near future, voting systems will stretch greatly beyond the national boundaries that currently contain them. The demands of ⿻ identity systems supporting some of the above suggest that while voting in new transnational configurations is imaginable, systems of voting are unlikely any time soon to truly reach global legitimacy. To truly reach that scope of diversity, we have to turn to the re-imagining of the thinnest of all the substrates for collaboration: market economies.